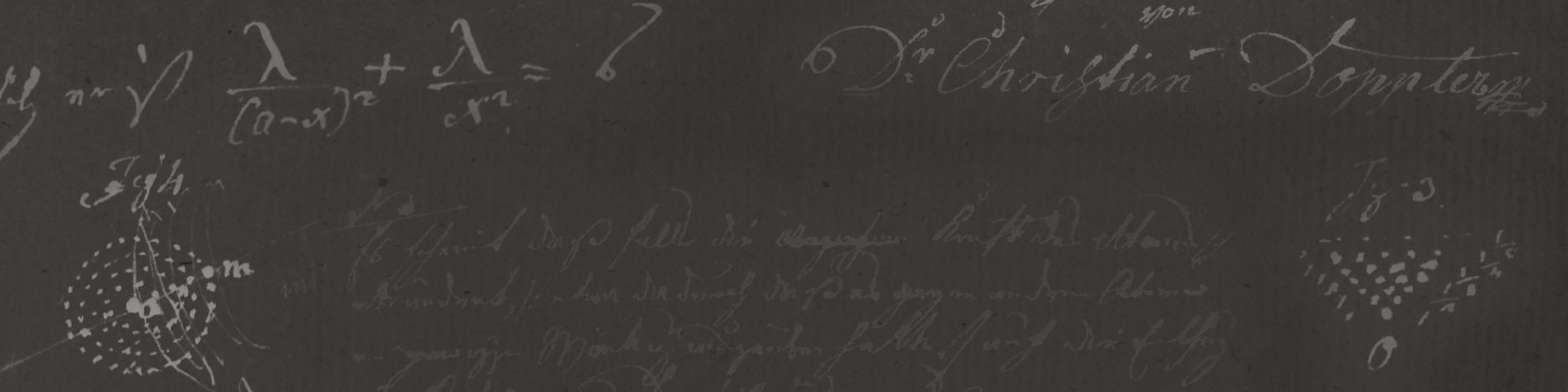Kulik, born in Lemberg (now Lviv), Galicia, was appointed professor of mathematics at the lyceum in Olomouc at the age of 21. 1816 Professor of Physics and Astronomy at the Lyceum in Graz. He received his doctorate in 1822 and was elected rector the following year in 1823. In 1826 he became professor of higher mathematics in Prague, where he lived until his death. He managed the library of the k. Böhm. Ges. der Wissenschaften and compiled a catalog of its holdings until 1835.
Kulik belonged to a generation of mathematicians that was more characteristic of the eighteenth century, which did not concern itself too much with the foundations of its work, since in this century of experimentation “results were produced in lavish abundance” (Struik, Dirk J.: Abriß der Geschichte der Mathematik, Berlin, 1967, p. 174) – “allez en avant, et la foi vous viendra” (Go forward, faith will follow), d’Alembert is said to have said.
Kulik created extensive factor tables and established a method for determining rational roots with the help of factor tables. He worked tirelessly on these until the end of his life. He wrote: “I possess a manuscript which contains the continuation of Burckard’s table from three million to 100 million on 4,212 closely written folio pages”. His manuscripts (8 volumes) have never been consulted in the archives of the Austrian Academy of Sciences in Vienna (information from Dr. Christa Binder, Vienna).
Jakob Kulik and Christian Doppler
At the meeting of the mathematical section on November 5, 1840, which Christian Doppler attended for the first time as an associate member of the Academy, Kulik read out an essay on a new analytical proof of the theorem of the parallelogram of forces. At the next meeting on December 3, 1840, Christian Doppler made critical remarks about the essay. Among other things, he said, “That if the sum of the squares of a function of two angles that add up to a right angle is equal to one, it does not follow that this function is the cosine of the angle, since several functions with this property can be given that are essentially different from the cosine.” Kulik replied that “Ref. would have exceeded the limits of a short essay if he had wanted to dwell longer on this theorem in order, as Littrow mentions somewhere, to fall into the error of some recent mathematicians who “cannot get to the point itself because of all the reasoning of the matter”.
Bolzano, who learns of this, comes to Doppler’s aid by stating in a substantial work that one can certainly linger longer on the reasons. “Whoever is content with the mere certainty that something is, and does not inquire into the objective reason why it is, has of course not yet awakened the scientific need”.
Against Kulik’s resistance, Bolzano managed to have Doppler’s first essay included in the publications of the Imperial Bohemian Academy in Prague in 1841. Academy. It had the long title: “Attempt at an analytical treatment of arbitrarily limited and composite lines, surfaces and solids, together with an application of this to various problems of descriptive and perspective geometry” and was intended to “generalize” the previous basic doctrines of analytical geometry.
Kulik, as director of the society, also gave a negative opinion on Doppler’s 1843 monograph entitled “Versuch einer Erweiterung der analytischen Geometrie auf Grundlage eines neu einzuführenden Algorithmus”. Nevertheless, Bolzano and Kreil endorsed the publication in the Abhandl. d. k. Böhm. Böhm. Ges. d. Wissenschaften V. Folge, 2nd volume, pp. 533-700, in Prague in 1843.
Dr. Peter Maria Schuster, 2017